Practical Endgame Test 6 / Attempts

White to play and win  

Diagram repeated for your convenience.

Arno: nice :-) Now the mutual damage plays at the top and bottom are miai, so in order to get tedomari I'd play the small hane on the left side. I think this way White wins. Sequence left for other people.

(after some thought) ... doh - maybe I am on the wrong track. Seems jigo as well.

dnerra: Arno, the hane on the left and saving the single stone in the center are absolutely miai, too. I won't comment on the correct solution as WillerZ is so close. But the two keywords are mentioned in Arno's remark...

Arno: I don't find a solution after Bill's diagram. I guess I'm blind. I'm waiting for the solution :-)

dnerra: Arno, you are not blind. You just blinked first, I suppose :)

unkx80: I believe there are two possible starting moves?

Bill: Ko threats aside, there is only one correct play, I believe.

unkx80: Okay I realised where my mistake is.

BTW, the discussion (quite interesting, IMO) has gotten so big that I think that I (or somebody) should move some of it to the Discussion page later.

WillerZ feels he may as well have a go:

White to play and win  

is 5 points in gote. is 4 points in gote. is 1 point reverse sente (counts as 2 gote). is 1 point sente (counts as 2 gote). is forced (10+ points in gote). 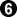is 2 points in gote. is two points in gote. 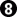is one point in sente (counts as 2 gote). is forced (2 points in gote). is one point in reverse sente (counts as 2 gote).

White to play and win  

Bill: Thanks, WillerZ. How do you respond to (instead of original )?
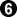WillerZ: Having thought some more, I wouldn't get into that situation in the first place. See below...

White to play and win  

WillerZ: I think this must be the correct way to answer .
is worth 2 (1 in sente).
is forced.
is worth 2 (2 in gote).
- are worth 2 (2 in gote).
is worth 1 (1 in reverse sente).
is worth 1 (1 in reverse sente).

Bill: I'm afraid I misled you, here. Using swing counting, as you are, you do double the size of reverse sente to compare them with gote. and are each worth the same as - . I have corrected my misstatement below. Sorry.

As for this variation, the result is a 24 to 24 jigo. Here is the accounting:

Black: Left side: 16
       Right side: 8
       Total:     24

White: Top side:     8
       Top right:    6
       Bottom right: 5
       Left side:    5
       Total:       24

In trying out variations starting after my on the top side, you don't really have to count. At that point all plays are worth the same, and the count is zero. So if Black gets the last play before the dame stage, the count will be unchanged for a jigo. If White gets the last play she wins. (Unless sombody makes a bad mistake, OC. ;-)) Note that White got the last such play in WillerZ's original solution.

Unless 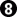is worth more than 2 points?

Bill: No, all plays are the same size. That's what makes it a problem. :-)

So far as I can see B has to get to a before W's response is worth more than 1 point in gote.

Bill: Continuing past a is also worth the same. ;-)

White to play and win  

WillerZ: it looks to me as though Black a and White b are worth the same as each other, 1 point with a 2 point followup. But the point to the right of a is (so far as I can see) 1 point with a 1 point followup, so defending against a is bigger than defending against the previous pushes. Yes?

Bill: Well, it's not the same, but the size is the same. Here is a tree for the plays on the top side, after Black plays to the edge. '/' shows a Black play, '\' shows a White play. White scores are negative.

                A
               / \
              B  -8
             / \
            C  -7
           / \
         -4  -6

The question is whether the move from C to -6 is bigger than the move from B to -7. To answer that let's find the counts at A, B, and C.

               -7
               / \
             -6  -8
             / \
           -5  -7
           / \
         -4  -6

From this it is plain that each play gains one point. (That does not mean that they are equivalent, which is part of the problem. :-))

Black makes jigo  

Bill: above ( here) is a mistake that lets Black make jigo. (I was wrong about there being only one correct response. It was OK to start with the sente on the right.)

Give it another try. :-)

White to play and win (if , )  

WillerZ: Okay. Thinking aloud, it doesn't matter whether B or W plays a, because the other will get the other 2 point gote at one of the b points (and vice versa). But White must play to keep parity with Black. So - 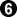are sente, forcing -. Interesting.

Bill: Bravo!

(Although 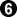and are miai. In fact, we can consider the whole thing as miai.)

White to play and win (11-17, pass)  

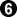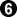is 1 point in reverse sente (counts as 2 gote). is zero points reverse sente, but has a 1 point sente followup -- a waste of a ko threat, but I don't see a ko happenning. is forced (1 point in gote). is setup for . is one point in gote. is forced (17 points in gote).

Final score is W:26, B:25

WillerZ: Correction to my above attempt.

White to play and win  

is worth 8 (4 points in reverse sente -- "a" is not black's sente any more).
is worth 5 (5 points in gote).
is worth 2 (1 point in reverse sente).
is worth 2 (1 point in sente).
is forced.
is worth 2 (2 points in gote).
is worth 2 (1 point in sente).
is forced.
is worth 2 (2 points in gote).
is forced.

Bill: WillerZ, is not reverse sente. But you do have to add the point at stake in the sente if Black plays . and have the same size. That's part of what makes this a problem. Which is better and when? :-)

White to play and win  

is forced.
is worth 2 (1 point in reverse sente).
White wins by 6 moku.

Bill: Look again. This is a 30 to 30 jigo.

WillerZ, your original solution is fine, except that Black can put up stouter resistance with . White can still win after in my diagram, but there is only one correct response. See if you can find it. I feel sure that you can. :-)