Hol Igor Solve Yose 1

Let us present separate games.

<0|-0.5 + b(0.5) + w(0.5) |0>

<0| B(3,0) + W(0,0)(B(2,0) +W(0,0)) |0>

<0| B(0,0)(B(0,0) +W(0,1))+ W(0,2)(B(0,0)+W(0,1))|0>

<29|B*(0,0)W*(0,0)B(2,0)(B(1,0)+W(0,0)(W*(0,0)+B(1,0))) + W*(0,0)B*(0,0)W(2,0)(W(1,0)+B(0,0)(B*(0,0) +W(1,0)))|27>

Reduction:

<0| -0.5 + b(0.5) + w(0.5) |0>

<0|B(3,0)+ W(0,0)( 1 + b(1) + w(1)) |0> =

<0| 2 + b(1) +w(1)(b(1) + w(1)) |0>

<0| B(0,0)( -0.5 + b(0.5)+w(0.5)) + W(0,2)(-0.5 +b(0.5) +w(0.5)) |0> = <0| -1.5 +b(1)(b(0.5)+w(0.5)) +w(1)(b(0.5)+w(0.5)) |0>

In the last game we will consider the branch after white played two moves. This corresponds to the position after .

The bags marked with circles and squares are <29| and |30>. The possible remaining positions are <0|1> after whites hanetsugi, <1| -0.5 + b*(0.5) +w(0.5) |0> after black's sagari.

The game is <29| W(0,1) + B(1,0)(-0.5 + b*(0.5) +w(0.5) |30> = <29| -0.25 + w(0.75) +b(0.75)(b*(0.5) + w(0.5))|30>

It is black's move now and the score is -0.5 + 2 -1.5 -1.25 = -1.25.

Let us rewrite the games again

<0|b(0.5) + w(0.5) |0>

<0| b(1) + w(1)(b(1)+w(1)|0>

<0| b(1)(b(0.5)+ w(0.5))+w(1)(b(0.5+w(0.5)) |0>

<0| b(0.75)(b*(0.5)+w(0.5)) + w(0.75) |0>

. Black takes 1 closing the game number 2.

. White takes 1 leaving game 3 open.

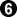. +0.75.

. The value of the game is the same as for the other two but it is a reverse sente. -0.5 .

. +0.5

. -0.5

The score is -1.25 + 1 -1 + 0.75 - 0.5 + 0.5 -0.5 = -1

White won, but after 3 the score was already well in white's favour.

If on the move 4 black plays in the game 3 then white gets 0.75 move at the bottom.

-1.25 + 1 -1 +1 -0.75 +0.5 -0.5 = -1